Demand Is Elastic Between Points

Nosotros use the point elasticity method when the changes in price and quantity demanded is very minor. Hence, information technology is easy to summate the elasticity at a indicate. And because changes are quite little, one can take the original price and quantity, as a base.

But, what to do when the change is substantial? 1 can neither have the initial toll nor the terminal cost every bit a base of operations. In such a example we utilize the arc elasticity method, wherein we use an boilerplate of both initial and final price.

What is Elasticity?

Elasticity is the responsiveness of the quantity demanded, as a outcome of a modify in price. In other words, it is the rate of change in the quantity demanded with respect to the charge per unit of change in cost.

When we talk near elasticity of demand we more often than not refer to price elasticity of demand. This is considering, due to their inverse human relationship, it gives a positive value. Further, when information technology comes to the measurement of elasticity of demand, there are 3 methods through which we tin calculate elasticity. These are:

Outlay method, or Total expenditure method.
Signal Method or Geometric Method
Arc method

Also Read: Difference Between Elastic and Inelastic Demand

In this mail service, we will talk over the differences between betoken and arc elasticity

Content: Betoken Vs Arc Elasticity

Comparison Chart
What is Point Elasticity?
What is Arc Elasticity?
Central Differences
Example
Conclusion

Comparing Chart

Basis for Comparison	Betoken Elasticity	Arc Elasticity
Meaning	Point Elasticity measures elasticity at a finite signal of the demand curve.	Arc Elasticity measures elasticity at the central point of an arc betwixt a pair of two points on the demand curve.
Introduced past	Marshall in 1890	Dalton in 1920
Measured when	Change is infinitesimally pocket-size	Change is finite (Detached)
Uses	Derivative of supply function	Deviation caliber
Per centum formula	Apply	Does not apply

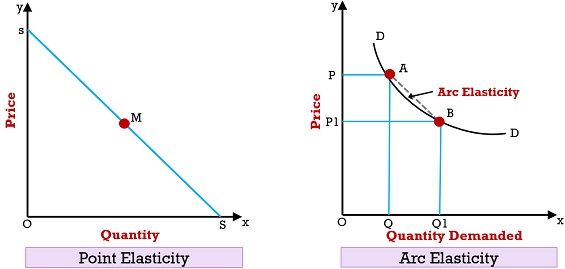
What is Point Elasticity?

Geometric measurement of price elasticity is possible through a method called the point elasticity method. It measures the demand at any point of the curve when the demand bend is linear.

As per this method, the price elasticity of demand of various points on the need curve shall be different.

What is meant by the term 'bespeak'?

In geometry, the term 'point' refers to something that occupies no space and dimensions.

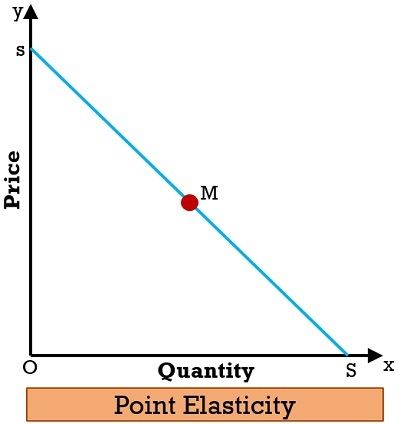Practically, bespeak elasticity is a measure of proportionate change in quantity demanded every bit a consequence of a very small proportionate change in the price. This concept is of import when the change in price and the resultant change in demand is infinitesimally pocket-sized.

Formula of Point Elasticity

One tin can utilize the formula given hereunder to measure elasticity:

Elasticity at different points on the Demand Bend

With the above graph nosotros take understood that at the mid-bespeak on the linear need curve, elasticity equals unity. However, at the higher points on the same curve, i.e. to the left of the mid-indicate, elasticity will exist greater than unity. Whilst, at lower points on the same curve, i.e. to the right of the midpoint, elasticity will be less than unity.

1 must note that, at the corner signal, i.due east. finish of the segment, elasticity equals zero. And, at the top, i.eastward. at the commencement of the segment, elasticity equals infinity.

In conclusion, every bit nosotros move from S towards s, elasticity keeps on increasing.

Likewise Read: Difference Between Demand and Quantity Demanded

What is Arc Elasticity?

Have you ever wondered, how can we measure elasticity between two points on the same need curve? So, we could do this through arc elasticity. For this, 1 has to calculate the averages of initial and final figures of price and quantity demanded.

Arc elasticity is the elasticity of a variable in relation to some other between two sets of points. This is used in the absenteeism of whatsoever general function to ascertain the relationship between 2 variables. We utilize this concept in 2 domains, i.e. mathematics and economics.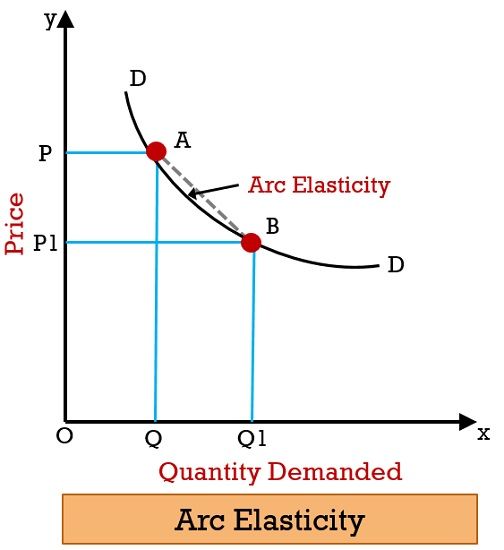

Further, we use arc elasticity to determine price elasticity over some part of the demand curve, instead of a single signal. In finer terms, with the aid of the arc method, we can compute elasticity over a range of prices.

Arc price elasticity of demand tends to measure the responsiveness of the quantity demanded in relation to the price of the product.

Points to Note

We measure the elasticity over the arc of the need bend on a graph.
It calculates the elasticity using the central point in between two points.
Information technology is used when at that place is a substantial alter in toll. Likewise, information technology conveys the aforementioned elasticity outcome, fifty-fifty if the price decreases or increases.

When we utilise the Arc elasticity method?

This method is used equally a measure where the discrete figures are bachelor for price and quantity.
When it is possible to set apart and compute incremental changes. It depicts the movement along a portion of the demand curve.

When we have to define the cost elasticity between two prices or say ii points on the demand curve, the question of base of operations cost pops up. That is which toll nosotros should consider as the base .As the elasticity computed by taking initial cost and quantity figures equally the base of operations will exist different from the one derived past taking new price and quantity figures.

Hence, to remove this confusion, nosotros employ an average of the ii prices and quantities every bit a base.

Formula of Arc Elasticity

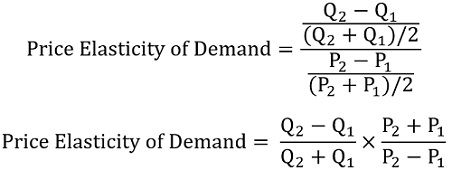
Where,

P1, Q1 represents the original price
P2, Q2 represents a new toll

Iii points should exist noted:

Arc elasticity is actually point elasticity at the fundamental point of the arc.
It becomes increasingly less accurate when movement is towards the arc's end.
The wider range, information technology makes the concept less useful.

As well Read: Departure Between Motility and Shift in Need Curve

Key Differences Between Point and Arc Elasticity

In the points given below, y'all volition find the differences between betoken and arc elasticity:

Price Elasticity of Need at a certain point on the need curve is the point elasticity of need. In contrast, Arc Elasticity refers to the elasticity amidst 2 points on the curve.
Marshall introduced the concept of point elasticity in the year 1890. Whereas Dalton coined the concept of arc elasticity in 1920.
The arc elasticity volition always autumn somewhere between pair of point elasticities, calculated at lower and college prices. Whereas Point Elasticity is the elasticity at a finite point on the curve.
In indicate elasticity, we make employ of derivatives in place of finite changes in price and quantity. While in arc elasticity, we employ a deviation caliber.
The percentage formula applies only in point elasticity. However, when at that place are finite changes in toll and quantity demanded is such that it relates to a extend over the need curve, then the percentage formula is modified is arc elasticity.
The point elasticity method of measuring elasticity is used in case the changes in price and quantity demanded are immeasurably small. This is for the reason that such a minute change indicates a virtual signal on the demand curve. Every bit against, if there are considerable changes we use the arc elasticity method. This is because nosotros are taking a detached move along an arc of the need curve.

Example of Arc Elasticity

Suppose we need to find an elasticity of demand for a product between:

P1 = ₹grand
P2 = ₹800
Q1 = 200 units
Q2 = 300 units

So, arc elasticity will fall somewhere point elasticity, calculated at lower and higher prices.

Conclusion

So, nosotros have understood that the difference between betoken and arc elasticity lies in the size of the modify in toll and quantity demanded. Based on our word we could say that point elasticity is a marginal concept.

Therefore, it is true for small-scale movements only from one bespeak to another forth the demand curve. Conversely, when the changes in cost and quantity are detached and large, we need to calculate elasticity over an arc of the demand curve.